Powder specimens of three different cubic crystals A, B, C, are analyzed with a Debye-Scherrer camera. It is known that the samples consist of fcc, bcc and diamond structures. The approximate positions of the first four diffraction rings in each sample are A) 42.2^o, 49.2 ^o, 72.0 ^o, 87.3^o, B) 28.8^o, 41.0^o, 50.8^o, 59.6^o, C) 42.8^o , 73.2^o, 89.0^o, 115.0^o
    a) Identify the crystal structures A, B and C.
    b) If the wavelentght of the incident X-ray beam is 1.5A, what is the length of the side of the conventional cubic cell in each case?
    c) If the diamond structure were replaced by a zinc-blende structure with a cubic unit cell of the same side, at what angles would the first four rings now occur?

2) Aschroft & Mermin P 6.2

3) Aschroft & Mermin P 6.5   

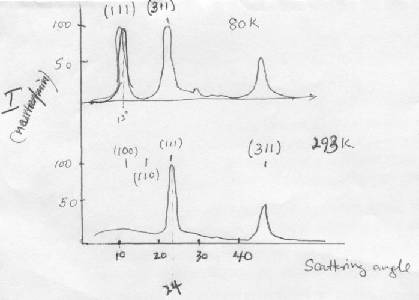
4) Neutron diffraction data for MnO differs drastically when the temperature is reduced below 120K. However, the X-ray data are nearly independent of the temperature showing same peaks as the 293 K neutron data and indicate a lattice constant a = 4.43 Å. (MnO has the same structure as rock salt.)
    a)      A MnO crystal does not show any Bragg reflections in the (100), direction either for X-rays or for Neutrons. Explain why? 
    b)      The diffraction peaks at two different temperatures for MnO crystal are shown in the figure. What is the approximate lattice constant at 80 K? (If you care: think about how does the state of the MnO differ at 80 K from its state at 293 K.)

5) Nacl crystallizes in fcc cubic lattice with a basis of Na and Cl ions separated by half the body diagonal of the cube. The atomic numbers of Na and Cl are 11 and 17 respectively. 

( Hint:  α = f _{C l}^_ / f _Na⁺  =17/11 )
    a)      Determine which X-ray reflections will be observed?
    b)      Of these which group will be strong and which group will be weak?

6) a) The concept of Bragg reflection is used to produce distributed Bragg reflectors (DBRs) widely used in optoelectronic devices. A DBR is made up of multilaryers of GaAs and A1As with refractive indices 3.6 and 2.96,  respectively. Show that if the optical thickness (nd) of each layer is λ/4, the structure acts as a mirror for normal incident light. 

    b) Born & Wolf show [1] that if light enters from GaAs into GaAs, separated by N period of A1As/GaAs quarter wave stacks the reflectivity is

What is the thickness of the stacks if a 99% reflectivity is required for a 0.8 µm GaAs laser?

References: [1] M.Born and E.Wolf, Optics, Pergamon Press , New york ,1987, page 69.